How to Calculate Power Factor Correction

Power Factor Correction (PFC) is a technique, which addresses this current-voltage mismatch in AC circuits. It uses capacitors which have an opposite reactive effect to inductors (common in motors and transformers), to bring the current and voltage back into alignment.

This improves the overall efficiency of the circuit, by reducing the reactive power component and lowering the current required for the same amount of real power transfer.

In DC circuit, power is a straightforward calculation of voltage times current (V*I). However the AC circuits are more complex because of a property called reactance. Unlike resistance, which simply opposes current flow, reactance can cause the current to be out of sync with the voltage.

This asynchronicity is captured by the power factor, a value between 0 and 1. A perfect “in sync” condition has a power factor of 1.

In AC circuit, the power used at any given moment is still calculated by multiplying voltage and current. But, unlike DC where these values are constant… both the voltage and current in AC circuits constantly change their strength and direction.

This is because, they alternate like a wave, following a sine wave pattern. The speed of this up- and- down cycle is determined by the frequency of the electricity source.

DC Circuits:

Imagine water flowing through a pipe (current) with constant pressure (voltage). The amount of water flowing out (power) is simply the pressure multiplied by the flow rate. That’s exactly how we calculate power in DC circuits: voltage (V) times current (I) equals power (V*I).

AC Circuits

Now lets picture a pulsating flow of water in the pipe, where pressure and flow constantly rise and fall together (like a wave). This is similar to how voltage and current change together in AC circuits. We can still calculate power, at any given instant by multiplying voltage and current, but…

The Out-of-Sync Culprit (Reactance):

Some AC circuit have components like coils, and transformers which act like a kink in the pipe. This kink disrupts the perfect alignment between pressure and flow (voltage and current).

In these cases, simply multiplying the voltage and current wont give us the exact amount of power being used (because some energy is being stored and released by the coils, not used).

The Role of the Phase Angle (Θ)

Engineers use a concept called the phase angle (Θ), to account for this kink. The phase angle tells us, how much the voltage and current are out of sync. By considering this angle, we can calculate the true average power which is being used in the AC circuit.

Remember from our discussion, on sinusoidal waveforms, the phase angle (Θ) tells us how much the current lags (falls behind) the voltage, measured in degrees. This lag happens because of reactive components in the circuit. In a perfect scenario with only resistance, the current and voltage are perfectly aligned, meaning they’re “in-phase” (Θ = 0°) since there’s no lag due to reactance.

In DC circuits, everything is straightforward… resistance simply opposes current flow. But, in AC circuits, things get more interesting. Components like inductor (coils, transformers) introduce a property called inductive reactance (X_L).

This reactance acts like a speed bump for the current, causing it to lag behind the voltage by 90 degrees. To account for both resistance (R) and inductive reactance (XL), we use a concept called impedance (Z).

Impedance, measured in ohms (Ω) just like resistance, is like the overall opposition an AC circuit presents to current flow. It considers the combined effect of resistance and reactance.

Take a look at this RL circuit:

Analyzing an Inductor and Resistor in Series (RL Circuit)

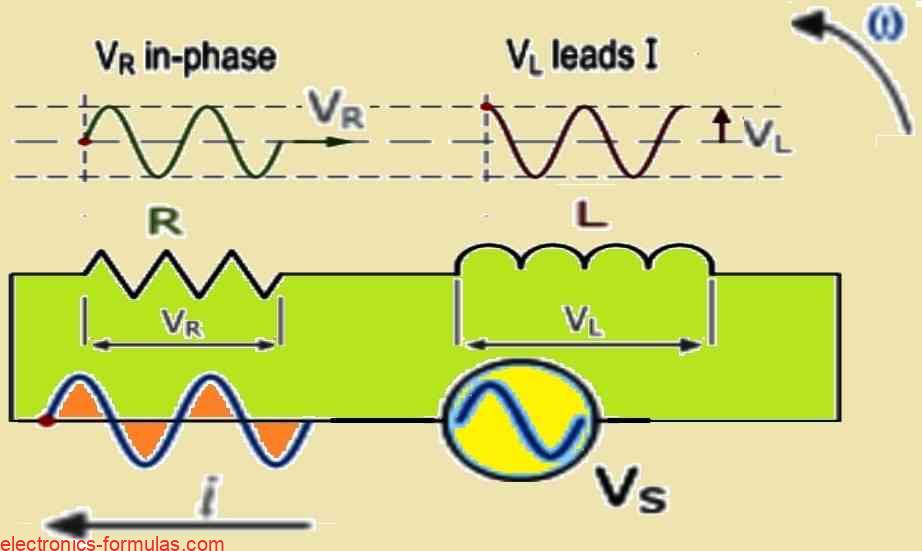
Because its a series circuit, the same current flows through both the resistor and inductor. This means, the voltage drop across the resistor (VR) is perfectly aligned (in-phase) with the current.

We can represent this on a phasor diagram, by placing the VR vector on the same axis as the current vector. However, the inductor creates a different scenario.

Its voltage drop (VL) is “out-of-sync” with the current. In this case, VL actually “peaks” (or reaches its maximum value) 90 degrees before the current peak (remember ELI – E=mLdI / dt).

This 90-degree lead is why the VL vector on a phasor diagram is drawn perpendicular to the current vector, pointing upwards.

On the phasor diagram, we will use the current vector as our starting point (reference). The voltage drops across the resistor (V_R) and inductor (V_L) will then be plotted based on their relative position to the current vector. As explained earlier, V_R will be in line with the current (0 degrees), while V_L will be drawn at a 90-degree angle “upward” because of the lag.

Phasor Diagram of an RL Circuit

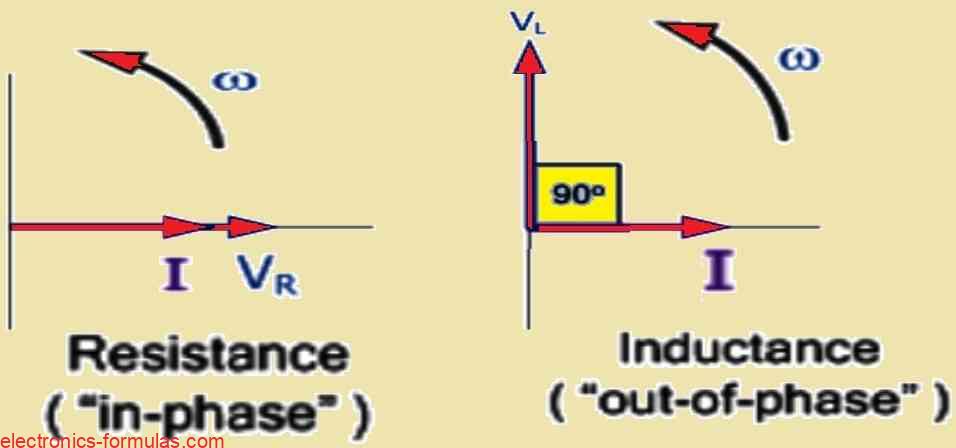
On a phasor diagram the resistor voltage (V_R) is plotted along the horizontal axis (also called the “real-axis”), because it’s in phase with the current.

The inductor voltage (V_L), however, lags the current by 90 degrees. So V_L is plotted on the vertical axis (also called the “imaginary axis”).

To find the total voltage (V_S) across the entire circuit, we need to combine these two voltage vectors, using the current as a reference point.

Since, V_R and V_L are perpendicular (form a right angle) due to their phase difference, we can use Pythagora’s theorem to calculate V_S. In simpler term, V_S is the hypotenuse of the right triangle, formed by V_R and V_L on the phasor diagram.

Visualizing Voltage Relationships in an RL Circuit (Phasor Diagram)

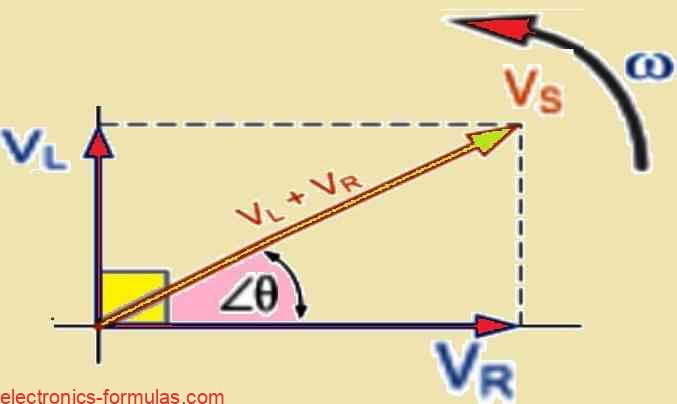
Here, the vector sum of V_R and V_L provides us with valuable information about the total voltage (V_S) in the series RL circuit.

Using Pythagoras theorem we can determine the magnitude of V_S: V_S² = V_R² + V_L²

This equation relates the squares of the individual voltage drops V_R across the resistor, and V_L across the inductor, to the squared magnitude of the total voltage (V_S).

Also the combined phasor diagram shows the phase angle (Θ) between V_S, and the current (i).

By utilizing the relative positions of V_R and V_L on the diagram and, their relationship with the current vector which acts as the reference, we can employ standard trigonometric function like sine [sin(Θ)], cosine [cos(Θ)], or tangent [tan(Θ)] to calculate the phase angle.

The phase angle quantifies the degree to which the total voltage (V_S) is out of sync with the current (i) in the RL circuit.

Solving a Power factor Correction Problem

We have an RL series circuit with the following parameters:

• Resistance (R) = 16 Ω
• Inductive Reactance (X_L) = 27 Ω

The circuit is carrying a current (I) of 6 amperes.

Please solve the following:

1. Find the supply voltage (V_S) across the entire circuit.
2. Find the phase angle (θ) between the supply voltage and the current.
3. Sketch a phasor diagram representing the voltage drops and their phase relationships.

Supply Voltage VS can be solved as follows:

• V_R = I * R = 6 * 16 = 96 V
• V_L = I * XL = 6 * 27 = 162 V
• V_S = √(V²_R + V²_L) = √96² + 162²
• V_S = 188.30 V RMS

We can confirm the above 188.30 V RMS results using the circuit impedances, as given below:

• Z = √(R² + X²_L) = √16² + 27² = 31.38 Ω
• V_S = I_S x Z = 6 * 31.38 = 188.30

To calculate the phase angle Θ, we can apply the Triganometry functions as below:

• cosΦ = V_R / V_S, sinΦ = V_L / V_S, tanΦ = V_L / V_R
• ∴ cosΦ = 96 / 188.30 = 0.50
• ∴ Φ = 60^o

From the above, we can draw the resultant phasor diagram, as given bellow:

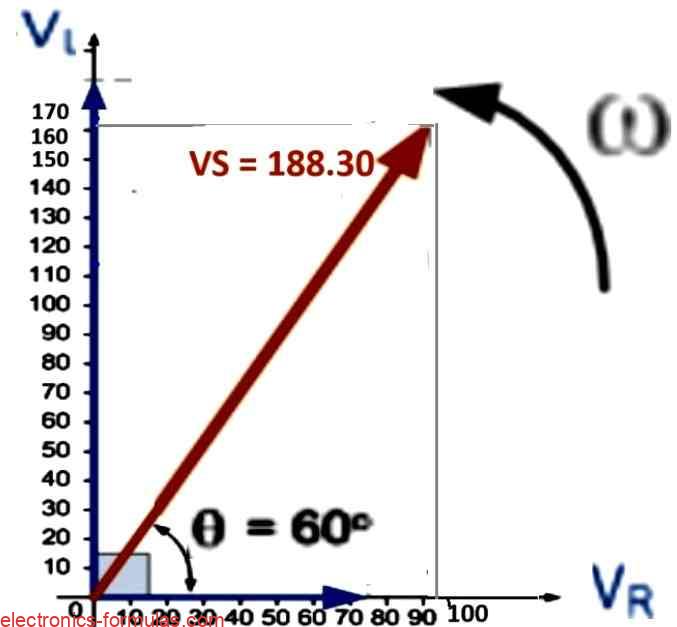
We found that the voltage drop across the resistor (representing real power) is 96 volts. While the voltage across the inductor (representing imaginary power) was 162 volts. Adding these two directly (96 V + 162 V), gives us 258 volts, which is a lot higher than the calculated total voltage of our 188.30 volts.

This difference arises because, 188.30 volts represents the phasor sum of the individual voltage drops. Phasor diagram account for the phase shift caused by the inductor, which doesnt directly contribute to real power consumption.

If you Know both the individual voltage drops (96V and 162 V) and the impedance values, you can further analyze the circuit to determine the actual power consumption (real, and imaginary), using appropriate calculation.

Power in an RL Circuit: Considering the Phase Shift

In circuit,s with reactive components like inductors or capacitors, the current (i) will not be perfectly aligned with the voltage.

This misalignment caused by reactance depend on the type of reactance. Capacitive reactance makes the current lead the voltage, while inductive reactance makes the current lag.

The power dissipated by the resistor, the ‘real power’ is measured in watts (W) and denoted by P.

Traditionally, it’s calculated using the formula P = I²R where I is the current and R is the total resistance.

However for AC circuits with reactance, calculating real power using just voltage (V_rms) and current (I_rms) requires an additional step. We need to consider, the phase angle (Θ) between voltage and current.

This angle reflects the degree of misalignment caused by the reactance.

To account for the phase shift we multiply the product of V_rms and I_rms by the cosine of the phase angle (cos(Θ)).

This gives us, the accurate formula for real power in an RL circuit:

Real Power, P = V_rms * I_rms * cos(Θ)

Power in a Resistive Circuit:

We saw earlier, that in a purely resistive circuit, the voltage and current are perfectly aligned or “in-phase.” This means there phase angle (Θ) is zero degrees (0°). Since the cosine of zero is one (cos(0°) = 1), multiplying the voltage (V), current (I), and cosine of the phase angle (cos(Θ)) is similar as simply multiplying voltage and current (V * I).

Therefore in a resistive circuit, calculating real power, using either V * I or I²R will gives you the same result.

For example, consider our previous example with a 15Ω resistor and a current of 5 ampere. The power dissipated by the resistor can be calculated using the formula:

Real Power (PR) = I²R

PR = (6 A)² * (16 Ω) = 576 watt

This is the same value we would obtain, by multiplying the voltage and current directly… assuming the voltage across the resistor is in-phase with the current.

This can also be expressed as:

P_R = V_R*I cos(Θ) = 96 x 6 x cos(Θ) = 576 watts

Understanding Power in Reactive Circuits:

In circuit containing reactive components like inductor or capacitors, the voltage and current wont be perfectly aligned. This misalignment, called “phase-shift,” leads to a concept called apparent power (S), measured in volt-amperes (VA).

Heres why:

• Apparent Power (VA): When voltage and current are out of sync, just multiplying their values (V * I) gives us the apparent power. However this does not directly translate to actual power consumption.

Inductive Circuits and Reactive Power:

Lets consider a purely inductive circuit where the current lags the voltage by 90 degrees (90°). Here, the product of voltage and current multiplied by the cosine of the phase angle (V * I * cos(90°)) becomes V * I * 0.

This calculation shows that, while theres no real power consumed by the inductor (no power loss), there’s still an “invisible” power exchange between the inductor and the source due to the phase shift. This “wattless” power is called reactive power (VAR), denoted by the symbol Q.

For an inductive circuit, this reactive power is represented by inductive reactive power (QL).

Capacitive Circuits and Reactive Power:

Similar to inductor, capacitors also exhibits a phase shift between voltage and current. In a purely capacitive circuit, the current leads the voltage by 90 degrees (90°). The reactive power for a capacitive load could be calculated using the formula: V * I * cos(-90°). However, this again results in V * I * 0.

This confirms, just like inductors, capacitors themselves don’t consume real power (no power loss), as PC = 0 watts. However there is still a reactive power exchange happening due to the phase difference.

To distinguish this “wattless” power in capacitive circuits, it is called capacitive reactive power (QC), which is measured in volt-amperes reactive (VAR). It’s important to note that the reactive power of a capacitance is defined as negative, hence the notation -QC. This negative sign signifies the opposite reactive power behavior compared to inductive circuit.

In our above example, the inductor constantly exchanges reactive power with the circuit, following a rhythm set by the frequency of the alternating current. This exchange could be described mathematically, by the following equation:

Q_L = I²X_L = 6² * 27 = 972 VAR

Because, pure inductors and capacitors cause a 90-degree phase-shift between voltage and current, we only consider the reactive component (the “vertical” part) of power.

This component is obtained by multiplying the voltage and current by the sine of the phase angle (Θ). However, since the sine of 90 degrees is 1, for purely reactive circuits, we can simply multiply the root mean square (rms) voltage and current values to find the reactive power. This is shown in the equation:

Q_L = I² * X_L = V * I * sin(Θ) = 162 * 6 * sin(90°) = 162 * 6 * 1 = 972 VAR

We can now observe, that the reactive power measured in Volt-Amperes Reactive (VAR), has the same magnitude as the real power (active power) in this case. However unlike real power, reactive power does not have an associated phase angle. This essentially means that reactive power always lies on the vertical axis of a power triangle at 90 degrees. So if we have this information, then:

P_R = I²R = 576 Watts

and Q_L = I²X_L = 6² * 27 = 972 VAR

With our understanding of Pythagoras’ theorem, and trigonometric function like sine, cosine, and tangent, we can now define a power triangle.

Understanding Power through the Power Triangle Equations

• VA² = W² + VAR² 🡢 S² = P² + Q²
• VA² = √(W² + VAR² ) 🡢 S² = √(P² + Q²)
• power factor, pf = cos(Φ) = Real Power / Apparent power
• pf = cos(Φ) = W / VA = P /S
• pf = sin(Φ) = VAR / VA = Q / S
• pf = tan(Φ) = VAR / W = Q / P
• P = VA cos(Φ)
• Q = VA sin(Φ)

Solving another Power Factor Correction Problem

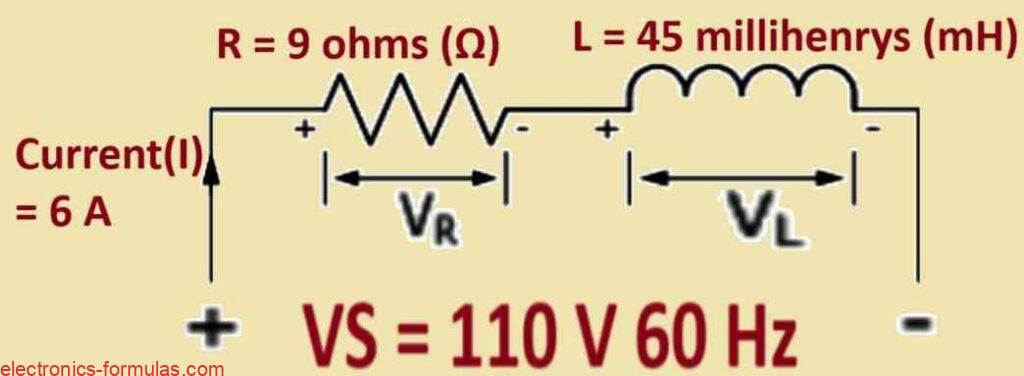
We have a coil, with a resistance of 9 ohms (Ω) and an inductance of 45 millihenrys (mH). When, this is connected to a 110 volts root-mean-square (rms) AC supply, at 60 Hertz (Hz), the circuit pulls a current of 6 Amps (A). Given this information, calculate the following:

1. The voltage across the resistor and inductor: How much voltage does each component experience?
2. The phase angle of the circuit: By what angle is the current lagging or leading the voltage?
3. The different power values: How much real, reactive, and apparent power do the circuit consume?

First we will calculate the impedances.

• X_L = 2πfL = 2 * 3.14 * 60 * 45 * 10^-3 = 16.95 Ω
• Z = √(R² + X²_L) = √9² + 16.95² = 19.19 Ω

Next, we will determine voltages across the resistor, V_R and inductor, V_L

• V_R = I * R = 6 * 9 = 54 V
• V_L = I * X_L = 6 * 16.95 = 101.7 V
• V_S = √(V²_R + V²_L) = √54² + 101.7² = 115.14 V

Now, let us calculate the phase angle of the circuit:

• cosΦ = V_R / V_S, sinΦ = V_L / V_S, tanΦ = V_L / V_R
• cosΦ = 54 / 115.14 = 0.46, therefore Φ = 62°

Now, let’s calculate the power of the circuit.

• Real Power (P) = V * I * cos(Φ) = 110 * 6 * cos(62°) = 303.6 watts
• Reactive Power Q = V * I * sin(Φ) = 110 * 6 * sin(62°) = 582 VAR
• Apparent Power S = V * I = 110 * 6 = 660 VA

The circuit draws approximately 690.84 Volt-Amperes (VA) of apparent power from the supply. This can be confirmed using the formula S = I²Z, where S is apparent power, I is current, and Z is impedance. In this case, calculating 6² × 19.19 ≈ 690.84 VA corroborates this value. Constructing a power triangle would visually represent this and confirm the apparent power.

Theres a catch, though. This high apparent power consumption (around 690.84 VA) of the RL circuit stems from a large phase angle (Θ). Remember the saying ‘ELI the ICE man’ – in this case, the voltage leads the current due to the inductance.

This large phase angle translates to a poor power factor of 0.46 (cosine of 62 degrees), indicating inefficient power transfer. To improve this we can strategically add a different type of reactance to the circuit. This reactance would have an opposite effect to the inductor, essentially canceling some of the unwanted inductive reactive power (about 582 VAR) used to maintain the coil’s magnetic field.

By introducing this compensating reactance, we can bring the power factor close to unity (ideal) and achieve a more efficient transfer of real-power.

Power factor tells you, how efficiently your coil is using the electricity it’s provided. Its like a measure of how much of the delivered power is actually doing real work. It’s calculated as the ratio of real power (in Watts), to apparent power (Volt-Amperes).

In our example the coil has a low power factor of 0.46 (303.6 W / 660 VA). This means that, a significant amount of the delivered power of around 356.4 VA (660 VA – 303.6 VA), is not contributing to useful work. The coil is actually using 660 VA of power to produce only 303.6 W of real power.

The culprit behind the coils low power factor is its inductive reactance, which acts like a positive opposition to efficient power use. To counter this, we can introduce capacitive reactance, that has the opposite effect. Think of it as adding a negative value to cancel out some of the positive one.

This technique, known as power factor correction, helps us bring the circuit’s overall power factor close to a perfect 1 (unity). By getting that number close to 1, we can ensure the coil is using most of the supplied electricity for real work, reducing wasted energy and lowering electricity cost.

Power Factor Correction

Power factor correction tackles the issue of a poor phase angle between voltage and current in the circuit, even-though the actual power usage (watts) remains unchanged. Remember that, pure reactance (like our coil’s inductance) doesnt consume real power, it just disrupts the flow.

By adding a capacitor in parallel with the coil (capacitance has an opposite reactive effect to inductance), we can decrease the phase angle (Θ). This decrease in phase angle improves the power factor, which ultimately reduces the total current (RMS current) that the circuit pulls from the power supply.

Power Factor in AC Circuits: A Balancing Act

The power factor in an AC circuit indicates, how efficiently electrical power is being used. It’s a ratio of 0 and 1, with 1 being the ideal scenario.

• The Influence of Inductive Loads: Inductive loads, for example like motors and transformers, tend to push the power factor down. In extreme cases (very heavy inductive loads), the power factor might reach around 0.2, but typically it won’t go any lower.
• Reactive Power Consumption: A power factor lower than 1 indicates the presence of reactive power. Reactive power doesnt do real work, but its presence affects how much current is drawn from the supply. As the power factor gets close to 0 (purely inductive circuit), reactive power consumption increases.
• The Goal: Reaching Unity Power Factor: Conversely, a power factor of exactly 1 signifies a perfect scenario. It means the circuit is purely resistive (just like an incandescent bulb) which consumes zero reactive-power. The voltage and current are perfectly in sync, resulting in a phase angle of 0 degrees. This ideal state is called “unity power factor.”

We now know, adding a capacitor in parallel with the coil can be a game-changer. Heres why:

Not only does the capacitor reduce the unwanted reactive power from the coil but it also helps decrease the total current drawn from the source!

Ideally, capacitor could completely eliminate the reactive power issue (100% compensation). However, in real-world applications, achieving a power factor correction between 95% and 98% (0.95 to 0.98) is often quite sufficient.

Remember the coil in our second problem with a power factor of 0.46? Lets find the capacitor value needed to improve it to 0.95.

The specific phase angle corresponding to a 0.95 power factor can be found using the cosine function (cos(0.95) = approximately 18.2 degrees).

• Tan (18.2^o) = Q / P = VAR / W = 0.329
• 0.329 = VAR / 303.6
• ∴ VAR = 303.6 * 0.329 = 99.88 VAR

The difference between these two VAR values (582 VAR – 99.88 VAR = 482.12 VAR), signifies the amount of reactive power reduction needed (in capacitive VAR) to improve the power factor.

Based on the calculated phase angle of 18.2°, we determine a specific reactive power value of 82.2 Volt-Ampere Reactive (VAR). This 82.2 VAR represent the capacitive VAR contribution required from the capacitor to achieve the target power factor.

In our example, the original uncorrected VAR value in the circuit was significantly higher at 582 VAR. The difference between these two VAR values (582 VAR – 99.88 VAR = 482.12 VAR), gives us the amount of reactive power reduction needed (in capacitive VAR) to improve the power factor.

To calculate this:

• VAR (capacitive) = Q_C = 482.12
• Q_C = 482.12 = V²_S / X_L = 115.14² / X_L
• ∴ X_L = 115.14² / 482.12 = 27.49 Ω

In our example, the capacitor requires a capacitive reactance of 27.49 Ω at the rated supply frequency. This reactance value is directly related to the capacitance of the capacitor through a formula. The formula used to calculate the capacitance (C) is:

X_C = 27.49 Ω = 1 / 2πfC

∴ C = 1 / 2 * 3.14 * 60 * 27.49 = 96 microfarads (μF)

As we saw above… correcting the power factor of the coil in our example from 0.5 to 0.95 requires connecting a capacitor in parallel with it. We calculated that a capacitor with a value of approximately 96 microfarads (μF) would be suitable for this purpose.

Now that the power factor correction is implemented, lets determine how this affects the real power delivered by the source.

Calculated Volt-Amperes After Power Factor Improvement

VA = √(W² + VAR²) = S = √(P² + Q²)

S = √(303.6² + 99.88²) = 319.60 VA

A power triangle can be constructed, to illustrate the before and after values for apparent power (VA or S) and reactive power (VAR or Q), as indicated below.

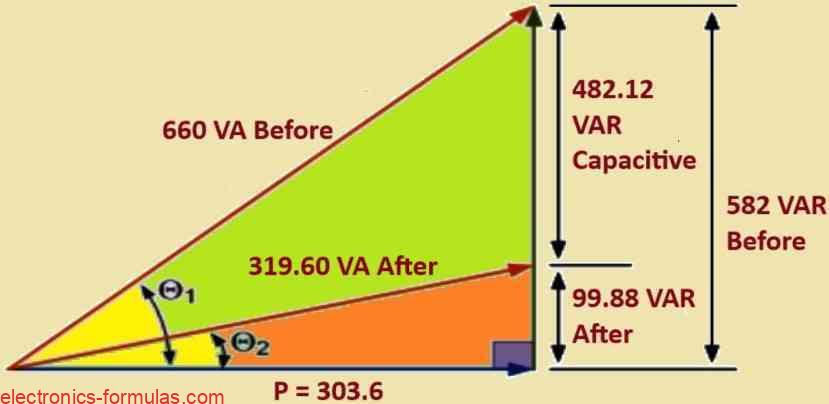
In the above figure, Θ1 = P / S1 = 303.6 / 660 = 0.46 pf (50%)

Θ2 = P / S2 = 303.6 / 319.60 = 0.95 pf (95%)

After the power factor correction, the circuits apparent power has significantly decreased, from 660 VA to 319.6 VA. This reduction allow us to calculate the new root-mean-square (rms) current supplied by the source.

S = V * I,   

therefore: I = S / V = 319.6/100 = 3.19 Amperes

Significant Improvement with Power Factor Correction:

Adding capacitor in parallel with the coil does not only improve its power factor – it produces real benefits! Here’s what we see:

• Power Factor Boost: The power factor jumps from a lower 0.5 to much better 0.95.
• Reduced Current Draw: The current drawn from the supply significantly decreases, coming down from 6 amperes to 3.19 amperes – a reduction of about 47%.

The Final Circuit Diagram with Power Factor Correction will be like this:

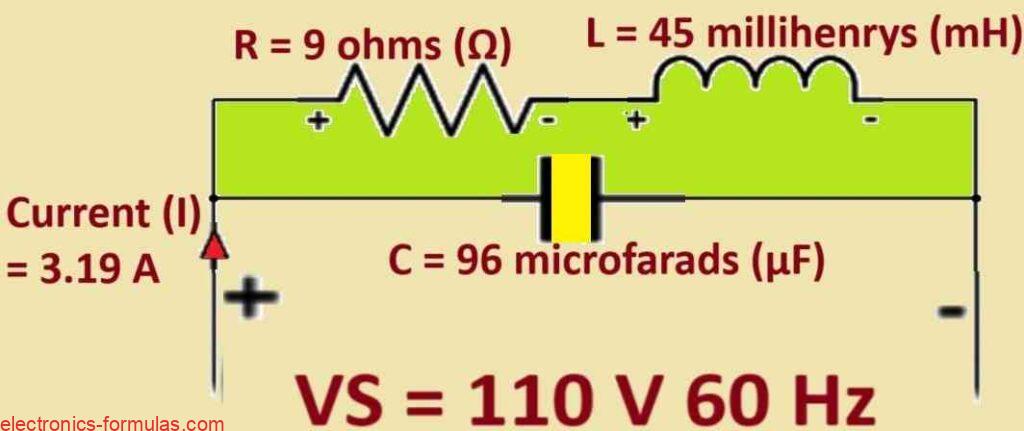
Conclusion

In most practical situations, just a single, a standard 100 μF capacitor, like the one we calculated would be sufficient for this example.

As we have learned in this tutorial, a lagging power factor caused by an inductive load leads to higher power losses in AC circuits.

By strategically adding a capacitor which acts as a capacitive reactive component, in parallel with the inductive load, we can reduce the phase difference between voltage and current. This technique called the “power-factor correction,” has two main benefits:

Improved Power Factor: It actually increases the power factor which is the ratio of real (active) power to the apparent power used by the circuit. This causes more efficient use of electricity.

Reduced Current Consumption: Due to this, the amount of current required from the source is reduced, which causes lower electricity bills and less strain on the power grid.

So finally, power factor correction enhances the circuit’s power quality by making it more efficient and reducing its burden on the electricity supply.

References: Power factor Correction

https://en.wikipedia.org/wiki/Power_factor